60 Doesnt Go Into 15

When it comes to division, we often expect neat and tidy results. However, there are times when the numbers just don't add up - or in this case, divide evenly. The phrase "60 doesn't go into 15" might seem simple, but it's a great way to introduce a fundamental concept in mathematics: division with remainders.

In this article, we'll delve into the world of division, explore what happens when numbers don't divide evenly, and discuss the importance of understanding remainders in various aspects of life.

Division 101: A Brief Refresher

Division is one of the four basic arithmetic operations, along with addition, subtraction, and multiplication. It's a way of sharing or grouping a certain quantity into equal parts or groups. When we divide one number by another, we're essentially asking how many times the divisor (the number by which we're dividing) fits into the dividend (the number being divided).

For example, 12 ÷ 4 = 3 because 4 fits into 12 exactly 3 times. However, not all divisions result in a whole number. That's where remainders come in.

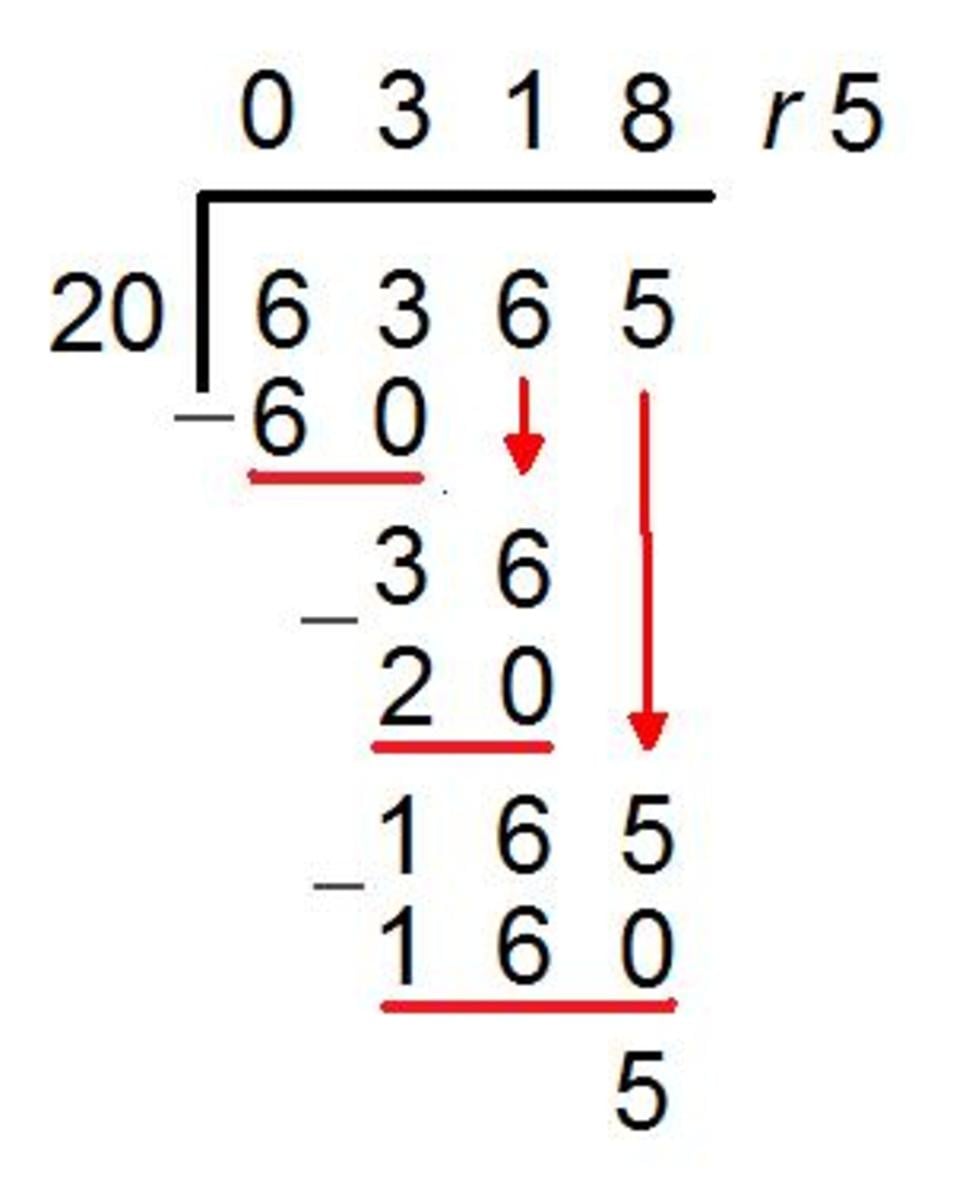
Understanding Remainders

A remainder is the amount left over when a number can't be divided evenly by another number. In the case of 60 ÷ 15, the result is 4 with a remainder of 0. But what if we were to divide 60 by 17? The result would be 3 with a remainder of 9, because 17 fits into 60 exactly 3 times, leaving 9 as the remainder.

Remainders are essential in various mathematical operations, such as:

Calculating fractions and decimals Determining the number of items that can't be divided evenly Solving problems involving time, money, and measurement

Real-World Applications of Remainders

Remainders might seem like a trivial concept, but they have numerous practical applications in everyday life. Here are a few examples:

Time and schedules: When planning a trip or scheduling appointments, you might need to calculate the remainder of hours, minutes, or seconds to determine the correct time. Finance and budgeting: Remainders can help you calculate change, determine the number of items that can be purchased with a certain amount of money, or figure out the remaining balance in your account. Cooking and measurement: When scaling recipes or converting between units of measurement, remainders can ensure that you have the correct quantities of ingredients.

Overcoming the "60 Doesn't Go Into 15" Mindset

It's essential to understand that division with remainders is not a limitation, but rather a natural part of mathematical operations. By embracing the concept of remainders, you'll become more proficient in various areas of mathematics and develop a deeper understanding of the world around you.

To overcome the "60 doesn't go into 15" mindset, try the following:

Practice division with remainders using real-world examples Use visual aids, such as blocks or diagrams, to represent division and remainders Explore different types of division, such as long division or synthetic division

Conclusion and Next Steps

In conclusion, the phrase "60 doesn't go into 15" is not a limitation, but rather a reminder that division with remainders is an essential part of mathematical operations. By understanding remainders, you'll become more proficient in various areas of mathematics and develop a deeper understanding of the world around you.

To further develop your understanding of division with remainders, we recommend the following next steps:

Practice division with remainders using real-world examples Explore different types of division, such as long division or synthetic division Apply your knowledge of remainders to everyday problems and situations

We hope this article has helped you understand the concept of division with remainders and how it applies to everyday life. Share your thoughts and experiences in the comments below, and don't hesitate to reach out if you have any questions or need further clarification.

Gallery of 60 Doesnt Go Into 15